What is the Difference Between Independent and Dependent Events Explained

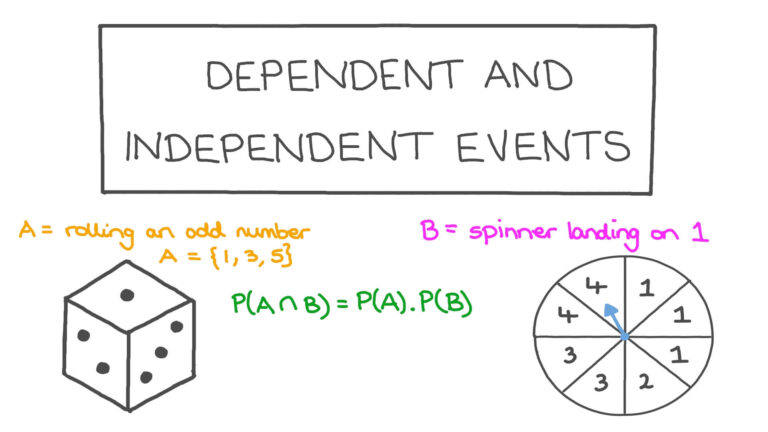
Understanding the disparity between independent and dependent events is crucial in the realm of probability theory. These concepts play a fundamental role in determining the likelihood of various outcomes in statistical analysis. Independent events occur when the outcome of one event does not impact the outcome of another, whereas dependent events rely on the outcome of one event affecting the outcome of the next. In this blog, we will delve into the nuanced differences between independent and dependent events, shedding light on how these notions are applied in real-world scenarios. By clarifying these distinctions, you will gain a deeper insight into the intricate workings of probability and sharpen your analytical skills.

Understanding the concepts of independent and dependent events is crucial in probability theory. Independent events are events where the occurrence of one event does not affect the occurrence of another. On the other hand, dependent events are events where the outcome of one event affects the outcome of the other.

Distinguishing Independent Events

Independent events are events that have no influence on each other. For example, flipping a coin and rolling a dice are independent events since the outcome of one event does not impact the outcome of the other. This independence makes calculating probabilities easier.

Understanding Dependent Events

Dependent events, in contrast, are events where the outcome of the first event affects the outcome of the second event. A common example is drawing cards from a deck without replacement. The probability of the second event is dependent on the outcome of the first drawing, making calculations more complex.

In real-life situations, understanding the difference between independent and dependent events can help in making better decisions and predictions.

Independent events are events whose occurrence or non-occurrence does not affect the probability of the other event happening. In simpler terms, the outcome of one event has no influence on the outcome of another event. This concept is crucial in probability theory and statistics.

Characteristics of Independent Events

1. Unrelated Outcomes: Independent events have outcomes that are not influenced by each other, ensuring that they are separate and unrelated.

2. Constant Probability: The likelihood of the second event occurring remains the same regardless of the outcome of the first event.

Dependent events refer to the situation where the outcome of one event affects the outcome of another event. In other words, the probability of the second event changes based on the outcome of the first event.

For instance, drawing two cards from a deck without replacement is an example of dependent events. The probability of drawing a second card of a certain type depends on what was drawn in the first draw.

To calculate the probability of dependent events, you multiply the probabilities of each event occurring in sequence. This differs from independent events where you multiply the probabilities of each event individually.

Understanding the dissimilarities between independent and dependent events is crucial in probability theory. Independent events are events where the outcome of one event does not impact the outcome of another. On the other hand, dependent events are events where the outcome of one event affects the outcome of another. The key differences lie in the relationship between the events and the probability calculations involved.

Probability Calculation

For independent events, the probability of both events happening is the product of their individual probabilities. In contrast, for dependent events, the probability of the second event is based on the outcome of the first event, leading to different calculations. Understanding these calculations is essential for accurate predictions.

Real-life Examples

Imagine flipping a fair coin twice. Each flip is an independent event because the outcome of the first flip does not affect the second flip. In contrast, drawing a colored ball from a bag without replacement involves dependent events since the probability changes with each draw. Real-life scenarios can illustrate these concepts vividly.

Independent events are occurrences where the outcome of one event does not affect the outcome of another. Here are some real-time examples:

Tossing a Coin

When tossing a fair coin, the result of one flip has no impact on the result of the next flip. This is a classic example of independent events.

Rolling a Dice

Rolling a dice multiple times, each roll is independent of the others. The probability of getting a certain number does not change with each roll. This demonstrates the concept of independence

Dependent events refer to occurrences where the outcome of one event directly affects the outcome of another. An example of dependent events is drawing cards from a deck without replacement. As each card is drawn, the probability of drawing certain cards changes based on the previous draws.

Example 1: Drawing Different Colored Balls

When drawing two balls from a bag without replacement, the probability of drawing a different colored ball on the second draw is impacted by the color of the first ball drawn.

Example 2: Choosing Marbles from a Jar

Consider a jar with red and blue marbles. If a red marble is drawn and not replaced, the probability of drawing another red marble decreases for the second draw.

• In this scenario, the events are dependent on each other, altering the likelihood of outcomes as the process continues.