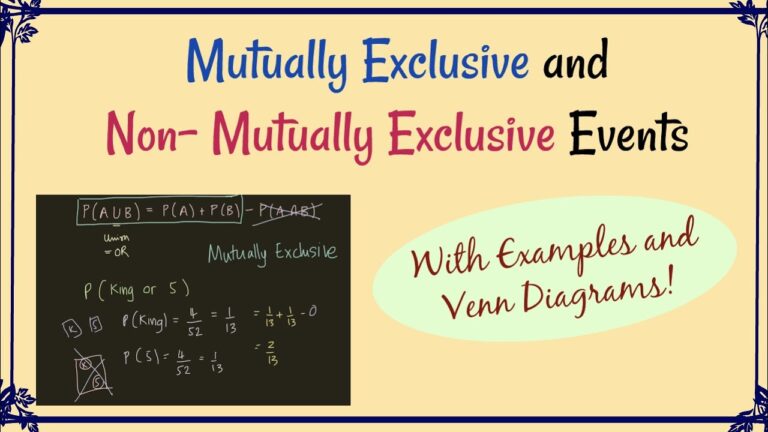Understanding non mutually exclusive events in probability is essential for grasping the intricacies of probability theory. When we talk about events in probability, the concept of “mutually exclusive” often arises, referring to events that cannot occur simultaneously. On the other hand, non mutually exclusive events are those that can occur at the same time. This distinction plays a significant role in calculating probabilities in various scenarios, ranging from simple to complex. In this ultimate guide, we will delve deep into the realm of non mutually exclusive events, unraveling the mystery and clarifying the concepts to empower you with a solid foundation in probability theory. Let’s embark on this enlightening journey together to unveil the secrets of non mutually exclusive events in probability.
Introduction to Non Mutually Exclusive Events
Non mutually exclusive events in probability refer to events that can occur independently of each other and do not impact the probability of the other event happening. Understanding the concept of non mutually exclusive events is crucial in the field of probability and statistics.
Key Characteristics of Non Mutually Exclusive Events
Non mutually exclusive events have the following key characteristics:
- Events that can occur simultaneously without affecting each other
- Events with no shared outcomes
- Events that have separate probabilities of occurring
Example of Non Mutually Exclusive Events
Consider the events of rolling a dice and flipping a coin. These two events are non mutually exclusive because the outcome of rolling the dice does not impact the outcome of flipping the coin, and vice versa.

Defining Non Mutually Exclusive Events in Probability
Non mutually exclusive events in probability refer to events that can occur independently of each other, where the occurrence of one event does not affect the probability of the other event happening. In simpler terms, these events can happen simultaneously or separately, and one event occurring does not impact the likelihood of the other event occurring.
Understanding Non Mutually Exclusive Events
When dealing with non mutually exclusive events, it’s crucial to note that their probabilities can be added together to calculate the total probability of either event occurring. This is in contrast to mutually exclusive events, where the probability of both events happening is zero.
For example, when rolling a six-sided die, the events of rolling an odd number and rolling a number greater than 3 are non mutually exclusive. The probability of rolling an odd number (1, 3, or 5) is 3/6, and the probability of rolling a number greater than 3 (4, 5, or 6) is also 3/6. The total probability of either event happening is the sum of these probabilities: 3/6 + 3/6 = 1.
Application in Real-life Scenarios
Non mutually exclusive events are prevalent in various real-life situations, such as weather forecasting, sports outcomes, and financial investments. Understanding the concept of non mutually exclusive events can aid in making better decisions based on probabilities and potential outcomes.
Understanding the Concepts of Probability
Probability is a crucial concept in mathematics that quantifies the likelihood of an event occurring. When it comes to non mutually exclusive events in probability, it refers to events that can occur independently of each other, without affecting the outcome of one another.
What is Non Mutually Exclusive Events in Probability
Non mutually exclusive events in probability refer to events where the occurrence of one event does not impact the occurrence of another. For example, the events of rolling a 2 on a dice and picking a red card from a deck of cards are non mutually exclusive.
Understanding the Calculation
To calculate the probability of non mutually exclusive events, you can use the addition rule. This rule states that the probability of either of two non mutually exclusive events occurring is equal to the sum of their separate probabilities.
For example, the probability of rolling a 2 or a 4 on a fair six-sided dice is 1/6 + 1/6 = 1/3.
Examples of Non Mutually Exclusive Events
In probability theory, non-mutually exclusive events are events that can occur simultaneously. These events have at least one outcome in common. Let’s explore some examples to better understand this concept.
Rainy Day and Traffic Jam
On a rainy day, the likelihood of a traffic jam increases. These two events, rainy weather and traffic congestion, are non-mutually exclusive as they can both happen simultaneously.
Defective Products in Batches
When manufacturing products in batches, the occurrence of defective products across multiple batches is a non-mutually exclusive event. One batch’s defects do not exclude the possibility of defects in another batch.
Image: Illustration of Non-Mutually Exclusive Events
Calculating Probabilities in Non Mutually Exclusive Events
When dealing with non-mutually exclusive events in probability, understanding how to calculate probabilities is essential. Unlike mutually exclusive events, where two events cannot occur simultaneously, non-mutually exclusive events can happen together. In such cases, probabilities are calculated differently to account for the overlapping possibilities.
Probability of Event A or Event B
To calculate the probability of either Event A or Event B occurring, the formula is P(A U B) = P(A) + P(B) – P(A n B), where P(A) represents the probability of Event A, P(B) is the probability of Event B, and P(A n B) is the probability of both events occurring simultaneously.
Using Real-World Examples
Imagine a scenario where you are drawing cards from a deck. The probability of drawing a red card or a face card can be calculated by adding the individual probabilities of drawing a red card and a face card, subtracting the probability of drawing a red face card since it’s counted twice.
Real-life Applications of Non Mutually Exclusive Events
Non-mutually exclusive events in probability are events that can occur independently of each other. Understanding this concept is crucial in various real-life scenarios where the occurrence of one event does not affect the probability of another event happening.
Stock Market Trading
Stock market trading is a prime example of non-mutually exclusive events. The rise or fall of one stock does not impact the rise or fall of another stock. This independence of events is a fundamental aspect of trading strategies.
Weather Forecasting
Weather forecasting utilizes non-mutually exclusive events to predict various weather patterns. The occurrence of rain in one region does not affect the chances of sunshine in another area. This independence allows meteorologists to make accurate predictions.
Tips and Tricks for Solving Non Mutually Exclusive Events
When dealing with non mutually exclusive events in probability, it’s essential to understand how they differ from mutually exclusive events. Here are some valuable tips and tricks to help you navigate this concept effectively:
Understanding Non Mutually Exclusive Events
Non mutually exclusive events are events that can occur simultaneously. To solve problems involving such events, you need to account for the overlap between them.
Tip 1: Use the Addition Rule
One of the fundamental tips for solving non mutually exclusive events is to apply the Addition Rule. This rule states that the probability of either of two non mutually exclusive events occurring is the sum of their individual probabilities minus the probability of their intersection.
Tip 2: Visual Representation
Creating a visual representation of non mutually exclusive events, such as Venn diagrams, can aid in better understanding the relationships between different events and their probabilities.
Frequently Asked Questions
-
- What are non mutually exclusive events in probability?
- Non mutually exclusive events in probability are events that can happen at the same time. In other words, the occurrence of one event does not affect the occurrence of the other event.
-
- Can you provide an example of non mutually exclusive events?
- A classic example of non mutually exclusive events is rolling a die and getting an odd number or rolling a die and getting a number greater than 4. Both events can happen independently.
-
- How do you calculate the probability of non mutually exclusive events?
- To calculate the probability of non mutually exclusive events, you add the individual probabilities of each event occurring and subtract the probability of both events happening at the same time to avoid double counting.
-
- What is the formula for calculating the probability of non mutually exclusive events?
- The formula for calculating the probability of non mutually exclusive events is given by P(A or B) = P(A) + P(B) – P(A and B), where P(A) and P(B) are the individual probabilities of events A and B, and P(A and B) is the probability of both events happening simultaneously.
-
- How are non mutually exclusive events different from mutually exclusive events?
- Non mutually exclusive events can occur simultaneously, while mutually exclusive events cannot happen at the same time. In the case of mutually exclusive events, the occurrence of one event excludes the possibility of the other event happening.
Unlocking the World of Non-Mutually Exclusive Events in Probability
As we conclude our journey through the intricate realm of non-mutually exclusive events in probability, we have demystified the concept that often perplexes many. By understanding that non-mutually exclusive events can occur independently, we have taken a significant step towards mastering the foundations of probability theory.
Through this ultimate guide, we have learned that non-mutually exclusive events have overlapping outcomes, opening up a plethora of possibilities in the world of probability calculations. Remember, the key takeaway is that the probability of at least one of the events happening can be found by adding their individual probabilities and subtracting the probability of their intersection.
So, armed with this newfound knowledge, embrace the complexities of non-mutually exclusive events with confidence and unravel the mystery of probability like never before!